Posted on December 14th, 2012 in Isaac Held's Blog
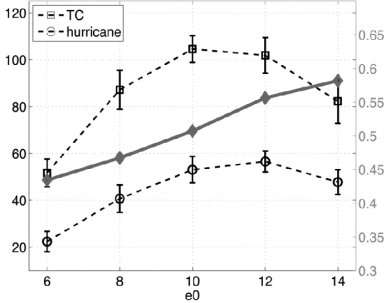 Globally integrated, annual mean tropical cyclone (TC) and hurricane frequency simulated in the global model described in Post #2, as a function of a parameter in the model’s sub-grid moist convection closure scheme, from Zhao etal 2012.
Globally integrated, annual mean tropical cyclone (TC) and hurricane frequency simulated in the global model described in Post #2, as a function of a parameter in the model’s sub-grid moist convection closure scheme, from Zhao etal 2012.
It is difficult to convey to non-specialists the degree to which climate models are based on firm physical theory on the one hand, or tuned (I actually prefer optimized) to fit observations on the other. Rather than try to provide a general overview, it is easier to provide examples. Here is one related to post #2 in which I described the simulation of hurricanes in an atmospheric model.
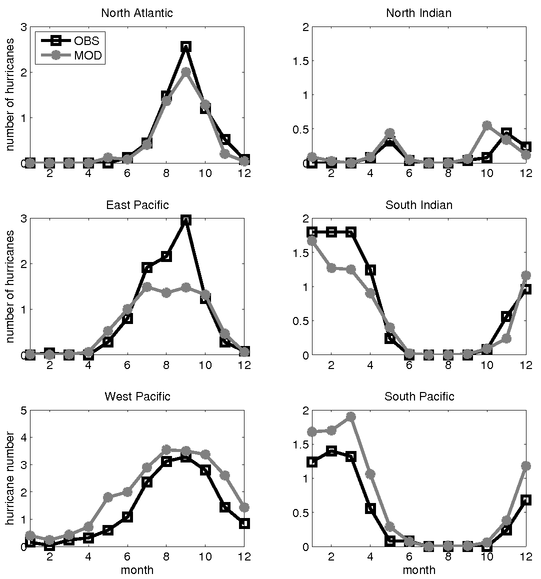
In that post you can find an animation of the model output and some comparisons with observations. Here’s a reprise of the figure on the seasonal cycle of hurricane frequency in the different ocean basins
This version of the model, which has about 50km horizontal resolution, seems to do a very good job at simulating the frequency of tropical cyclones – (max winds > 17m/s) and the fraction of these storms of hurricane strength (> 33 m/s), but does not simulate very strong (cat 3-5) storms, although the intensity distribution looks better if you look at minimum pressure rather than maximum winds. I have been impressed by the quality of this simulation and similar simulations in other models. We also have a 25 km version that produces quite similar results. Yet many in the tropical cyclone research community remain skeptical that a model with 25-50km grid size can simulate the physics of TC formation.
When we first described these results in Zhao et al 2009 we knew very little about their sensitivity to model parameters. We still don’t, because the model is computationally expensive. Why work with a model that is so resource-consuming? It’s a tension that is always present in climate modeling: do you use increasing computer resources to create higher resolution models with the idea that improvements in the simulation will make the higher computational burden worthwhile, or do you stop with a more modest model that allows you to vary parameters systematically? If one is interested in phenomena that are difficult to resolve in typical global models, such as tropical cyclones. the choice is pretty obvious — you need to push the resolution to build a case for the credibility of the simulations.
A recent paper Zhao etal 2012 describes the sensitivity of TC and hurricane frequency in this model to two parameters — one of these is shown in the figure at the top. The parameter is part of the sub-grid closure scheme for moist convection in the model. The plot shows the average number of TCs per year on the whole globe (one of the dashed lines), as well as the number of TCs of hurricane strength (the other dashed line). For convenience it also redundantly shows the fraction of TCs that are of hurricane strength (solid line with scale on the right). We run a 20 year simulation for each of 5 values of
; the “error bars” are the standard deviation of the 20 yearly values . As
increases the total number of TCs and hurricanes first increases and then decreases. The fraction of TCs that reach hurricane strength, a crude measure of average intensity, increases monotonically with
.
In the tropics a lot of the vertical transport takes place in plumes generated by moist gravitational instability that extend from near the surface to just beneath the tropopause. The dominant horizontal scale of these plumes might be of the order of one or a few kilometers — although direct simulation of the turbulent entrainment into and detrainment out of these plumes, which affects their buoyancy, requires still smaller scales. If you don’t have a sub-grid scale convection scheme in your model, “plumes” will still occur but in a distorted way on the scale of the model grid. In reality convection occurs even though the average conditions over, say, a 50 km square are not conducive to the generation of gravitational instability — due to spatial variability on smaller scales. Closure schemes for moist convection are based on an explicit or implicit picture of what is going on within a grid box that determines if convective plumes are triggered, how much mass is transported to the upper troposphere within the plumes, etc. In the case of the closure scheme used here, when is small deep convection occurs relatively easily; when it is large the convection is more inhibited.
It happens that the value we chose to use was , close to the value that produces the maximum number of TCs. The main reason for this choice was the model’s top-of-atmosphere energy balance, which is sensitive to
, varying by more than 10 W/m2 over this range of values, due mostly to changes in low cloud. It is hard to find other ways of counteracting such large changes to rebalance the model. And the model becomes quite noisy on the grid scale at the higher values of
examined. It was these considerations, rather than systematic examination of storm statistics vs.
, on which the initial choice of this parameter value was based.
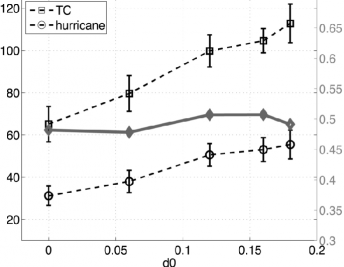 The other parameter we have examined directly controls the grid-scale noise in the model, especially in the tropics. (The horizontal flow on each model level can be decomposed into rotational and divergent components — the parameter
The other parameter we have examined directly controls the grid-scale noise in the model, especially in the tropics. (The horizontal flow on each model level can be decomposed into rotational and divergent components — the parameter controls the strength of the damping of the divergent component only, which affects the flow primarily in the tropics.)
As increases this damping of small scales increases and one might expect the number of TCs, which after all are only marginally resolved by the grid, to decrease, But the opposite occurs — the number of TCs increases as the small scale damping increases in strength. The intensity as measured by the fraction of TCs that become hurricanes stays about the same. In fact it is hard to find anything in the simulation that is affected by this parameter other than the number of TCs that the model generates — and explicit measures of how noisy the model tropics is close to the grid scale. Our interpretation of this result is that it is the competition for a resource (the evaporation of water at the surface) that is the key — if you have too may little nascent disturbances trying to grab their share it becomes difficult for vortices of TC strength to form. We think that this dependence on the noise level is also responsible for the reduction in storm counts at large
. (Other effects are dominant at small
.)
These dependencies are still under investigation. But it should be clear that the kind of results displayed in Post #2 are not entirely “first principles” simulations of TC statistics, and the picture could change as we move to finer and finer resolution, especially to the point of resolving some of the deep plumes dominating moist convective turbulence in the tropics. Are we justified in using this model as a tool to ask how hurricane statistics respond to warmer SSTs/increasing greenhouse gases?
I put a lot of weight on results such as the seasonal cycle figure above. The simulations hold together remarkably well. Nothing has been done to try to tune these seasonal cycles. I don’t know how to quantify my level of confidence based on the quality of the simulations, but I would argue that tropical cyclone projections with this class of model should be taken seriously despite legitimate concerns about dependence on the treatment of sub-grid scale processes.
[The views expressed on this blog are in no sense official positions of the Geophysical Fluid Dynamics Laboratory, the National Oceanic and Atmospheric Administration, or the Department of Commerce.]



Thank you for this insight in how TC simulations are done. I think one should always be aware that those models are not perfect, that many things in there are missing and the resolution is still very rough. But on the other hand we must decide now what to do about rising GHG levels and we don’t have the time to wait for the perfect model and a 100% certain answer. And at least we can see e.g. from the distribution of hurricane counts in the figures in your post, that the models are doing at least some things not too bad.
So IMHO we should treat models as what they are: They are the best we have for future climate prediction and therefore we should use their results (with care) in our decisions (and I don’t see any reasonable alternative to that).
I agree with your comments, although with regard to the sensitivity of tropical cyclones to warming, in addition to global models such as this we also have regional models and theoretical insights into the genesis and potential intensity of tropical cyclones to work with.