Posted on December 7th, 2011 in Isaac Held's Blog
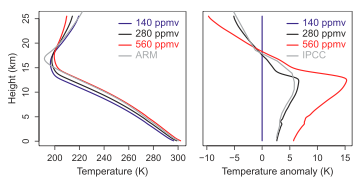 Results from a high resolution model of horizontally homogeneous radiative-convective equilibrium, Romps 2011. Left: equilibrium temperature profiles for 3 values of CO2 compared to an observed tropical profile. Right: the temperature differences compared to the response to doubling CO2 in an ensemble of CMIP3 global climate models.
Results from a high resolution model of horizontally homogeneous radiative-convective equilibrium, Romps 2011. Left: equilibrium temperature profiles for 3 values of CO2 compared to an observed tropical profile. Right: the temperature differences compared to the response to doubling CO2 in an ensemble of CMIP3 global climate models.
As a moist parcel of air ascends it cools as it expands and does work against the rest of the atmosphere. If this were the only thing going on, the temperature of the parcel would decrease at 9.8K/km. But once the water vapor in the parcel reaches saturation some of this vapor condenses and releases its latent heat, compensating for some of the cooling (you get about 45K of warming from latent heat release when a typical parcel rises from the tropical surface to the upper troposphere). A warmer parcel contains more water vapor when it becomes saturated, so it condenses more vapor as it rises, and temperature decreases with height more slowly. That is, the moist adiabatic lapse rate, , decreases with warming.
To say something about the warming of the tropical atmosphere, rather than that of a moist adiabat, we need to argue that the tropical troposphere is close to a moist adiabat and remains close as it warms. The upper troposphere will then warm more than the lower troposphere. This is precisely what happens in our global climate models. The consistency or inconsistency of this prediction with observations, particularly the Microwave Sounding Unit (MSU) temperatures, is a long-standing and important issue A failure of the upper troposphere to warm as much as anticpated by this simple argument would signal a destabilization of the tropics — rising parcels would experience a larger density difference with their environment, creating more intense vertical accelerations — affecting all tropical phenomena involving deep convection. I like to refer to warming following the moist adiabat as the most “conservative” possible — having the least impact on tropical meteorology.
(One sometimes sees the argument that a consequence of smaller upper tropospheric warming in the tropics would be lower climate sensitivity, since a large fraction of water vapor feedback originates in this region, and the large vapor increase could not occur without the temperature increase. But this is not the case, because of the cancellation between negative lapse rate and positive water vapor feedbacks produced by upper tropospheric warming. In fact, the negative lapse rate feedback is generally the larger of the two, so a weaker upper level tropical warming would probably increase climate sensitivity a bit, holding everything else fixed.) [This statement continues to be a source of confusion — sorry — see discussion in the comments. The water vapor feedback that I am referring to here is the part associated with the lapse rate change, if relative humidity is fixed, after subtracting off the part due to uniform warming of the troposphere (IH- Jan 26, 2013)]
The tropical atmosphere, and models of moist radiative-convective equilibrium, are dominated by concentrated saturated updrafts taking up a small fraction of the total area, with the rest of the flow experiencing very slow compensating subsidence. The behavior of such a skewed flow field can be counterintuitive. The picture that most of us have, I think, is that within the convective updrafts themselves the temperature profile takes its moist adiabatic value; this profile is then communicated efficiently to the rest of the tropics, since the atmosphere is unable to maintain substantial horizontal temperature gradients within the tropics. Horizontal gradients in pressure and temperature, above the boundary layer, are flattened by wave propagation rather than by mixing, a fundamentally different process than the homogenization of entropy in a dry convecting layer.
I remain somewhat confused as to how best to translate this picture into a scaling argument for how hard one has to push the tropical atmosphere to create a given departure from the moist adiabat. Arguments of the type summarized in Emanuel, Neelin, and Bretherton, 1994 suggest that attempts to alter the free tropospheric temperature profile will modify temperatures by a rather indirect path — heating perturbations will modify the circulation in a way that then modifies the temperature and humidity of the air near the surface, which finally puts you on a different moist adiabat. The calculations by Kuang 2010 in which a dynamic model of radiative-convective equilibrium is perturbed systematically with different heat (and moisture) sources suggests that this is the path of least resistance for a convecting atmosphere, but that there are other possibilities as well — I need to understand this paper better.
It doesn’t take much of a departure form an adiabat to be dynamically significant. A 3K temperature difference between parcel and environment averaged over a height
naively produces an acceleration of
and velocities at the top of the convective layer of
— which is larger than vertical motions observed in tropical convection. But it is not that easy to relate vertical motions quantitatively to departures from an adiabat in the tropics. There are subtleties in the definition of the moist adiabat itself associated with what happens to the condensate — temperatures are slightly different if the condensate is retained by the parcel, in which case its heat capacity must also be taken into account, or if the condensate falls out immediately, in which case we refer to the “pseudo-adiabatic” lapse rate. Real parcels are somewhere between these two extremes. You also needs to worry about the latent heat of fusion when ice forms, the presence of supercooled water making it tricky to predict when this transition to ice occurs. In addition, when computing the density difference between a rising parcel and its environment, and the associated vertical accelerations, you must account for the “condensate loading” — the pressures associated with the suspension of the condensate within the rising parcel. Finally, if a parcel entrains some dry environmental air as it rises, it has less latent heat to release per unit mass, and its temperatures will fall faster with height than the temperature profile generated by an undilute parcel.
Several of these effects can be of the order of a degree or two — they are big enough to matter when trying to estimate the magnitude of the departure of the tropical atmosphere from a moist adiabat — the CAPE (Convective Available Potential Energy) of the tropics (see, for example, Xu and Emanuel 1989 and Williams and Renno 1993). But none of them are large enough to alter the expectation that the tropical atmosphere will roughly follow a moist adiabat as it warms. One of these effects would have to change by an O(1) amount (doubling or halving its amplitude) in response to a 2K warming, say, to have a substantial effect on the sensitivity of lapse rates to warming, but why should that happen?
This conclusion is confirmed by high resolution models of horizontally homogeneous radiative-convective equilibrium, every one of which, to my knowledge, predicts a warming profile that is more or less moist adiabatic. The figure at the top is from Romps 2011, mentioned in the last post as well, which has 200m horizontal resolution. The figure on the left shows the model’s equilibrated temperature profiles at three values of CO2 along with an observed tropical profile, while the panel on the right shows the changes in temperature in this model along with an average over CMIP3 global models (with grid sizes roughly 100 times larger). The profile of temperature change is essentially identical. There is actually a rather large fractional increase in CAPE and increase in the magnitude of vertical motions as the climate warms in these simulations, but this increase is nowhere near large enough to compensate for the upper level maximum in warming.
(The fact that the overall amplitude of the warming for doubled CO2 is nearly identical in the ensemble mean of GCMs and in this cloud resolving model is a coincidence — it is the vertical profile of the temperature change that I am focusing on here. The variety of results on sensitivity with cloud resolving models of radiative-convective equilibrium is as large as that obtained with GCMs, due primarily to differing cloud feedbacks associated with differences in organization of convection. These high resolution simulations are not necessarily more relevant to nature than GCMs, due to the idealized geometry and small domain, absence of rotation etc. The value of these dynamic radiative convective equilibrium models, even in these small idealized domains, is in testing our undersatanding of moist convection. )
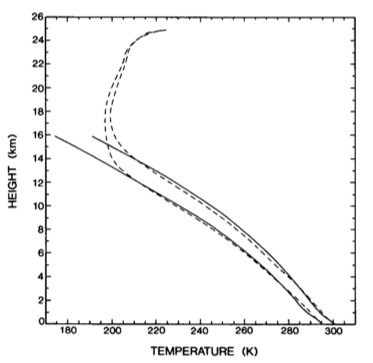
The change in CO2 itself has very little to do with this moist adiabatic response; you get essentially the same temperature response if you just just prescribe and then warm the surface temperature. Here, for example, is an early attempt at a dynamic radiative-convective model, from Held et al, 1993, for a 5K surface warming with fixed CO2 (the solid lines are moist adiabats):
A dramatic change in convective organization can change the relevant moist adiabat constraining tropical temperatures. The self-aggregation transition described in the previous post, is a good example. The aggregated state is warmer by several degrees averaged over the troposphere than the state with more homogeneous convection, because the near surface relative humidity is higher in the region in which the convection is occurring — due, in turn, to increased winds and evaporation. (Thanks to Caroline Muller for confirming this for me.)
As one moves upwards and convection peters out, there is presumably some potential to change local temperatures with local perturbations in ozone or aerosols, perhaps above 12 km or so. But below that, if you are trying to change the relationship between surface and tropospheric warming, it seems that one is better off trying to change the relevant moist adiabat, by changing low level humidities or temperatures in the convecting regions, rather than creating huge departures from a moist adiabatic profile.
In addition to the analyses of MSU temperatures (which I won’t try to summarize here) , there is other relevlant observational work that we need to focus on. One is the study of Allen and Sherwood 2008, using thermal wind balance to relate trends in the vertical gradient of the zonal winds to trends in the north-south temperature gradient The thermal wind equation is very accurate for zonal mean winds throughout the atmosphere, a simple consequence of the assumptions that the mean state of the atmosphere is in hydrostatic balance and that winds are in geostrophic balance. One can use an even more accurate relation, gradient wind balance, but given the large uncertainties in atmospheric warming trends, thermal wind balance is certainly accurate enough. I would like to see more attention on this use of wind trends, since these are totally independent of the temperature measurements, satellite or radiosonde.
Another study deserving attention is Johnson and Xie 2010 which argues that one can look at the distribution of deep convection in the tropics and rule out a trend towards overall destabilization. The temperature (and associated water vapor content) of near surface air has to reach a certain threshold for this air to rise close to the tropopause — currently 26-28C. If the atmosphere follows a moist adiabat as it warms, this critical temperature will increase along with the surface warming. If the upper troposphere does not keep up, this critical temperature would not increase as fast as the surface temperature itself, favoring more widespread convection — which, according to the paper, is not observed. This is another paper that I have to think about more carefully.
[The views expressed on this blog are in no sense official positions of the Geophysical Fluid Dynamics Laboratory, the National Oceanic and Atmospheric Administration, or the Department of Commerce.]



Isaac,
I have a question about the paragraph related to the self-aggregation transition:
—-
The aggregated state is warmer by several degrees averaged over the troposphere than the state with more homogeneous convection, because the near surface relative humidity is higher in the region in which the convection is occurring — due, in turn, to increased winds and evaporation. (Thanks to Caroline Muller for confirming this for me.)
—
Is the increased evaporation that maintains a higher RH mainly in the non-convective region or in the convective region? If the former were the case, then is it possible that a higher RH in the convective region be maintained by a more efficient lower-level moisture transport from the non-convective region where the net surface radiation also favors enhanced evaporation?
I haven’t looked at this in detail, but thinking about it in response to your question (changing my story a bit), I am not sure that you need any change in evaporation to get this result –the moisture convergence due to the circulation generated by the localized convection is probably enough.
Isaac,
Thanks for the post. Another effect that can change the ratio of upper tropospheric to surface warming on a moist adiabat is the relative humidity near the surface. I think a 3-4%/K decrease in humidity is enough to wipe out any upper tropospheric amplification. However, the required changes in winds to keep the evaporation reasonable are large, and probably fit in with your characterization of needing to change other quantities by O(1) to significantly affect the warming profile.
Right — that’s what’s happening in the self-aggregation transition. It is hard for me to imagine this large a reduction in tropical mean near surface humidity with warming — but one only has to get this reduction in the convecting regions.
Following up a bit — the SSMI measurements of vertically integrated water over the oceans and the comparison with GCMs — ie, Fig, 1 in
Soden at el 2005 — pretty much rule out any big problem in the simulations with low level humidity trends over the oceans — so the trend in the tropical ocean mean moist entropy of the sub-cloud layer must be about right as well, so there seems to be no reason to believe that one is getting on the wrong moist adiabat. It is hard to see how these measurements could be consistent with an increase in moisture that was just half of the Clausius-Clapeyron value. At a minimum, one would need to argue for a distinction between tropical mean trends and trends in the regions of deep convection.
Isaac – Among unsettled issues is the change in upper tropospheric humidity with warming – specific humdity increases, but there is conflicting information as to whether this increase is sufficient to maintain a near-constant relative humidity (RH – e.g. Minschwaner and Dessler 2004 as compared with Soden et al 2005). To what extent would a reduction in RH affect the change in the moist adiabatic lapse rate as a function of warming?
The Minschwawaner and Dessler study is of interannual variability. In ENSO cycles the tropics follow the moist adiabat pretty closely, as one would naively expect based on the tropical mean surface ocean warming — and models capture this temperature response very well. (This is one of the reasons why the possibility of a departure from moist adiabatic warming in the trend would be so curious, the atmospheric dynamics involved being fast enough that it should not care whether the warming is due to ENSO or slowly evolving forcing.) So I do not see this study as hinting that the modest RH variations observed could be responsible for departures from a moist adiabatic response.
Here’s another independent method for looking at tropical tropospheric temperature changes.
“The Threshold Sea Surface Temperature Condition for Tropical Cyclogenesis”
https://journals.ametsoc.org/doi/abs/10.1175/JCLI-D-10-05006.1?journalCode=clim
No temporal trends observed for the threshold cyclone temperature (the many caveats in the paper noted). So the debate rages.
I have a question. A scan of the literature seems to suggest that most of the focus is on bring observation in line with models. Can you point me to published work that does the opposite. Analysing the possible shortcomings of the models playing “what if” games to try to bring the models in line with the observations. Or are these experiments just not possible?
I think of the temperature threshold for tropical cyclogenesis as the same thing as the threshold for convection to the tropopause, so, based on a reading of the abstract alone, it looks like there may be a conflict between this paper and Johnson and Xie. It would be interesting to sort this out.
As for manipulating GCMs to conform to some specified data set, it depends a lot on the observations you are talking about and the “stiffness” of the model in that respect. There are a host of papers that take this approach, but mostly in the model development rather than climate change literature, in which the target is just the mean climatology. I am sure that you can just google the double ITCZ problem to see people thinking about how to fix this common model shortcoming, as an example. With regard to fitting trends, there is a tension between wanting to use observed trends to evaluate model physics and wanting to project into the future with a model that is simulating past trends optimally. This can be a source of confusion when different modeling groups take different approaches .
The tropical lapse rate’s response to warming is pretty stiff, as I was trying to emphasize in this post. I think the most plausible route to getting a different result for the lapse rate trend is through the addition of other forcing agents, other than well-mixed greenhouse gases, acting near the tropopause. If you are convinced that your model is deficient in this regard that’s what I would play with.
Dear Dr. Held,
This was a very interesting article and helped clarify some issues for me.
If you have the time I would like to ask some questions that may be helpful to me and others. If you don’t have time I would certainly understand.
1) How certain are you that a weaker than expected hotspot would lead to a slightly higher climate sensitivity? Is it not possible that this could go in various ways? That is, is there not significant uncertainty about all of the feedbacks impacted by tropical upper tropospheric warming, i.e. water vapour feedback, lapse rate feedback, and cloud feedback, e.g. Colman 2001 (Climate Dynamics, “On the vertical extent of atmospheric feedbacks”).
2) On the thermal wind equation, I wonder if you know what happened to a paper by Pielke et al. 2008, discussed at https://pielkeclimatesci.wordpress.com/2009/01/28/submitted-paper-assessment-of-temperature-trends-in-the-troposphere-deduced-from-thermal-winds-by-pielke-sr-et-al/
3) In this post you have not mentioned a recent paper by Fu, Manabe & Johanson (2011, “On the warming in the tropical upper troposphere: Models versus observations”, GRL). These authors conclude “While satellite MSU/AMSU observations generally support GCM results with tropical deep‐layer tropospheric warming faster than surface, it is
evident that the AR4 GCMs exaggerate the increase in static stability between tropical middle and upper troposphere
during the last three decades.” Do you agree with these authors? Do you regard this as a potentially controversial result?
4) There is an argument from Lindzen (2007, “Taking greenhouse warming seriously”, E&E) that has never been discussed in the literature, as far as I am aware. The argument is laid out in section 2. Lindzen himself evidently regards it as still valid today (e.g. he has cited it in the recent Lindzen/Choi 2011 paper). I assume you would regard the argument as invalid. Would you mind giving your thoughts on what he gets wrong?
With best regards,
Alex Harvey
PS. I am NOT the same Alexander Harvey who has posted here in the past!
Alex,
1) I was referring to the sum of water vapor and lapse rate feedbacks. If relative humidity is unchanged, the sum of these feedbacks is small but negative in response to an increase in tropical upper tropospheric temperatures. This is just a statement about radiative transfer. I am also quite confident that relative humidity will not change much ( I haven’t discussed the source of this confidence on this blog yet.) As usual, clouds are the major source of uncertainty, and I did not intend to include them in this comment. I guess the bigger point is that if the tropical lapse rate changes in such a way as to destabilize the tropical atmosphere a lot (which I am still skeptical of), this says that we are missing something important, the implications of which are hard to predict until we understand it.
2) I have no knowledge of that work
3) I’ll return to Fu et al in a future post
4) thanks for pointing out this Lindzen paper, which I was not aware of. Section 2 of that paper is partly devoted to an explanation for what the greenhouse effect is — this is entirely standard and exactly the explanation that I always use. The rest of this section discusses some radiosonde-based trends in lapse rate and the implications of those being correct. It might be better to return to this issue after the post that I promised above.
Dear Dr. Held,
On point 2, Pielke et al. 2008 (submitted), the material may have been published in:
What Do Observational Datasets Say about Modeled Tropospheric Temperature Trends since 1979?
John R. Christy 1,*, Benjamin Herman 2, Roger Pielke, Sr. 3, Philip Klotzbach 4, Richard T. McNider 1, Justin J. Hnilo 1, Roy W. Spencer 1, Thomas Chase 3 and David Douglass 5
Remote Sensing 2010, 2, 2148-2169; doi:10.3390/rs2092148
After some discussion of the thermal wind equation, they conclude that “…these trends calculated from the TWE, as applied for AS08 and here (C10), using the current radiosonde coverage and observational limitations (consistency, accuracy, etc.) do not produce results reliable enough for studies such as ours. In particular, AS08 and C10, with TLT trends of +0.29 and +0.28 °C decade−1 are almost three times that of the mean of the directly measured systems, and are values that are, in our view, simply not consistent with the countervailing, directly-measured evidence.”
On the remaining points I thank you for your reply and look forward to reading more about this at your blog in the future.
Isaac – You state that the sum of water vapor and lapse rate feedbacks is net negative. Is this a different conclusion from Soden and Held 2008, or is the difference related mainly to the fact that your above statement refers only to the tropical upper tropospheric temperature?
Fred,
Since the lapse rate feedback wins over water vapor feedback in the global mean, and both are dominated by the tropics, I was assuming that this was true of the tropics in isolation. Typically, temperatures win because water vapor is weakened by upper level clouds — in the clear sky it would be a much closer compensation. If you haven’t seen it you might be interested in the nice discussion of water vapor feedback in Ingram, 2010.
Isaac,
Your statement “the lapse rate feedback wins over water vapor feedback in the global mean” confuses me. I thought that the rough numbers were that the water vapor feedback about doubles the climate sensitivity from the no-feedback value and then the lapse rate feedback takes about half of that back, leaving about a 1.5X-or-so increase in the climate sensitivity. Is this wrong…Or is your statement about the “global mean” only applied to the upper troposphere.
Could you clarify how all of these fit together?
Sorry for being unclear. I am thinking of the water vapor feedback as divided into two parts (let’s assume that relative humidity is fixed for simplicity). The first part is due to the increase in vapor that would occur with uniform warming of the troposphere. The second part is due to the additional vapor you get, per unit surface warming, if the lapse rate decreases (and the upper troposphere warms more that the surface). It is this second part, the water vapor feedback associated with the lapse rate change, that compensates for part, but not all, of the lapse rate feedack. So when comparing two models with more or less fixed relative humidity, I expect the one with more upper tropospheric warming to be less sensitive — ignoring clouds etc. The total water vapor feedback, including the part due to uniform warming, is larger than the lapse rate feedback, as you describe. I’ll be coming back to this in an upcoming post.
Thanks, Isaac. That makes more sense to me now! And, I just want to say that I really appreciate your blog…It had fallen off my “radar screen” for a few months and I am glad that I remembered to come back to it again.
Judging from the other comments, it looks like you are providing a great resource both for others working in the field to exchange ideas but also for some of us who are not climate scientists, but who have the necessary background to at least sort-of follow more technically-oriented discussions than are available on most blogs on the topic, to learn a lot! (In my case, I am a physicist for which reading about climate science has become a hobby.)
Isaac – Thanks for the clarification, because I had been puzzled too. Incidentally, in my initial comment, I misstated the date of the relevant paper I wanted to mention. It was Soden and Held 2006, not 2008
Is there anyplace not behind a paywall that one can learn about the basics of cloud-resolving models? I was surprised to learn that this one didn’t include diurnal changes in radiation and other influences. Some convective processes are part of large organized circulation (the Hadley cell for example) that is much larger in scale than this model. Do we have any idea how far from a convective “tower” (if that is the right term) subsistence usually occurs? Rising air follows a moist adiabat once it has reached the lower condensation level, but subsiding air should follow a dry adiabat. I don’t understand how mixing under these circumstances produces a tropical atmosphere which follows a moist adiabat.
To access papers, I usually start by looking at author home pages and then use google scholar to find copies outside of the firewall if there is one, and if that fails I just contact the author. Possible starting points might be homepages for Chris Bretherton (UWash) , Bjorn Stevens (Max Planck, Hamburg) , Graeme Stephens (JPL), Zhiming Kuang (Harvard). I am not aware of a review paper on the subject of dynamic radiative convective equilibrium models specifically.
Many very high resolution models include diurnal cycles, but typically in more realistic settings — see, for example, here .
Radiative convective models in small domains, no matter what the resolution, do not simulate the spatial inhomogeneities and convective structures that occur in the tropics, and should not be thought of as providing quantitative simulations of tropically-averaged clouds (or relative humidity for that matter). Most research with these kinds of models are not this idealized, of course, and involve attempts to simulation specific phenomenon. I find the homogeneous radiative convective framework useful for testing ideas, despite its idealization.
The picture of the compensating subsidence in the tropics putting the atmosphere in these regions on a dry adiabat is not correct. This is part of what I referred to as the counterintuitive behavior of a flow with such dramatic skewness in its vertical motion. The upward motion is confined to such a small area, and the subsidence so slow, that one cannot ignore the radiative cooling in the subsiding air. In fact the waves that homogenize temperatures in the horizontal are so fast we think of the subsidence rate as being determined by the requirement of consistency between the radiative cooling rate and need for the parcel’s temperature, as it descends, to follow the moist adiabat determined by rising plumes. This kind of balance is formalized in what we refer to as weak-temperature gradient dynamics — see, for example, Bretherton and Sobel, 2002. In this theory, one assumes that the subsidence is spread uniformly over the entire tropics!
Isaac writes “But once the water vapor in the parcel reaches saturation some of this vapor condenses and releases its latent heat, compensating for some of the cooling (you get about 45K of warming from latent heat release when a typical parcel rises from the tropical surface to the upper troposphere).”
Water vapour condensing is accompanied by a decrease in volume but this never seems to be mentioned in descriptions of the moist adiabat. Yours is no exception… is there a reason its ignored? And how does it figure into the explanation?
It’s a small effect, much smaller in the atmosphere than the expansion due to the reduction in pressure as the air rises, but the reduction in volume at a given pressure due to condensation is included in accurate computations of adiabatic lapse rates.
Also water vapour is “lighter than air” and so doesn’t require parcels of air to be rising for the water vapour itself to be rising. If the water vapour were to be considered to be rising in its own right what implications would that have? Obviously air will come along for the ride (and have its own convection related reasons to rise too) but most explanations I’ve seen appear to treat the “parcel” as the driver of the process when that doesn’t feel right to me.
I think I need a really good reference to our current understanding of these issues. Any suggestions?
This question does not relate to condensation, so we can assume that there is none for simplicity. Picking a small piece of fluid — a parcel — there is then no way for the center of mass of the vapor in this parcel to move with respect to the rest of the air molecules except by molecular diffusion — in fact, you can take that relative motion to be the definition of molecular diffusion. You have to picture the water molecules as locked into the parcel along with the other molecules due to the small mean free path between collisions — ie, because molecular diffusion is small. The water molecules cannot just up and decide to do their own thing. You have to be up above 100km for the density to decrease enough so that this relative motion of different species becomes significant. An undergraduate level atmospheric thermodynamics textbook might be a good place to start. I don’t teach undergraduates, so I am not a good person to ask about introductory texts — perhaps the one by Grant Petty — I haven’t looked at how that text discusses this particular issue.
This is a very common error that unfortunately developed some traction on the web in the context of the ozone hole and CFC transport into the stratosphere — “aren’t they heavier than the rest of the air molecules, so wouldn’t they stay close to the surface?” Dispelling this notion is usually the first order of business in any class on atmospheric chemistry — which suggests that an intro textbook on atmospheric chemistry might also be good place to look for a discussion of this.
So mixing trumps diffusion. OK fair enough. Thanks for taking the time to respond Isaac.
Hi Isaac. I know very little about tropical dynamics, but have a half-thought question related to the tropical lapse rate: Is there a simple argument for why the relative humidity over land is expected to remain fixed? I could imagine that it might change, due to soil moisture, which might be affected by changing moisture convergence in the atmospheric column above, or changing runoff. I could also imagine that deep convection takes place preferentially over land, and so the relative humidity over land might be very important for setting the tropical lapse rate.
Joe — great question. What determines the moist entropy of the boundary layer air over land where convection occurs? In the climate change context, can the entropy over land increase more than it increases over the oceans? See Joshi et al 2008 and, in particular the conference presentation by Byrne and O’Gorman, who argue that the changes in entropy should be the same — that warming is larger over continents because the entropy increases are the same as over the oceans but relative humidities are lower. I need to think about this more myself.